Radian measure practice

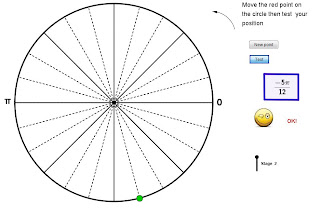
This is one of the simpler of a huge number of elegant GeoGebra creations from Daniel Mentrard. (Those available in HTML rather than Java are here.) You're given an angle which is some integer multiple of pi/12 and asked to place it on the appropriate place on a circle. You then push the test button to see if you've placed it correctly. You can choose between "Stage 1" and "Stage 2" problems. Stage 1 has only positive angles. Stage 2 includes negative angles.

Simplifying Expressions with Integer Exponents

This applet from Larry Green provides practice in simplifying expressions with integer exponents.

Transforming f into g

Note: If you're not seeing both graphics windows on GeoGebraTube, you can download the applet and run it on your desktop.

In this applet (inspired by Steve Phelps' What's My Rule series) you select a parent function, f(x),  from among 10 possibilities and click a button to indicate the maximum number of transformations (up to 4) that you'd like to have performed on f to produce a new function g. You see only one point on g, but you can move the corresponding point on f, to determine the relationship between the two. When you believe you have found the parameters that describe the transformation, you can show the graph of the transformed function that your parameters create and see if the lone point moves along it.

Here's a Java version of the applet.

Ferris Wheel

This is a full-featured and beautifully designed GeoGebra applet from John Golden that allows students to practice fitting parameters to a cosine function which models the height of a ferris wheel car above the ground as a function of time. You can watch the ferris wheel spin as the height curve is generated and it provides an endless source of practice since you can always generate a new ferris wheel.

Napier's Gift

I was inspired by the first chapter of Eli Maor's e:The Story of a Number to create this GeoGebra applet designed to help the user discover how to simplify the process of finding a quotient by subtracting exponents. The idea is both to introduce students to logarithms (though they are never mentioned explicitly) and to help students understand why they were so heralded when they were introduced. The applet is also posted on GeoGebraTube.

Daylight Hours Explorer

This Daylight Hours Explorer is from a fabulous collection of astronomy simulations and animations at the University of Nebraska at Lincoln. Exploring hours of daylight over the course of a year at various locations on the earth is a great way for students to think carefully about parameters of trig functions.

Visualizing Radian Measure

Ted Coe's Unwrapping a Circle applet on GeoGebra Tube is a visually powerful way of demonstrating what a radian is. I've also posted a slight modification of the applet optimized for use as a demonstration.

Indiana Puzzle Quilt

This applet was inspired by a quilt square my mother-in-law made. It provides a nice way of visualizing the  the sum of an infinite geometric series.
Check out the pattern in some real quilts, too!

Update 1/5/2017: See the Snail's Trail Quilt Square

Spinning Out Sine and Cosine

A nice animation from the Wolfram Demonstrations Project showing the relationship between a circle and the graphs of sine and cosine. I like the way the cosine graph is show on its side. I'm going to try using it (after editing it in Mathematica to remove the sine and cosine labels) as an introduction both to the unit circle and the trig graphs. It looks like a good candidate for the technique of showing an animation with no commentary and then asking for student questions, writing them on the board, and asking for answers to/discussion of any of the questions.

Constructing the Area of a Circle

A beautifully designed applet that walks you through finding the area of a circle by dividing it up into sectors and rearranging them to form a shape which becomes a rectangle as the number of sectors goes to infinity. I recommend using it in conjunction with Steven Strogatz's fabulous article, "Take it to the Limit". (If you like this, you might also like this applet which approaches the same problem in a slightly different way.)

An actually fun fraction game!

Refraction is truly a game that teaches mathematical concepts. It's fun. And whether you know the underlying concepts well or not, you'll stick with it because, as my ten-year-old said, "it's addicting." I came across it through a series of twitter posts from Dan Meyer (@ddymeyer). How could I not try it when he was saying things like "The summer Olympics are coming up, right? I'm probably going to medal in this fraction game" and "Yeah, I should go pro at this game. I've found my calling."  While it's obviously meant to teach fraction concepts, it also requires lots of spatial thinking. In the first couple of rounds, there's nothing that looks like a fraction, you just figure out how to use the tools provided to bend light beams in the right way to power a ship. In typical video game fashion, you're introduced to harder puzzles and new tools that help you solve them as you move through the levels.

My ten-year old was watching me play an early level, and despite the fact that her math-teacher mom was doing something with fractions all over the screen, she was intrigued and asked to play. She kept at it until we called her to dinner in half-an-hour or so, and she went back to it after dinner. Later, after she'd made it far into the game, she watched me working on a level that was a little higher than she had reached (because, as I said, it's actually fun and I wanted to play, too!) and had ideas that she really wanted to try, so I gave it back and let her at it. It was interesting to listen to her talk to herself as she worked through the puzzles. She was whispering things like ("the denominators have to be the same" and "yeah, 8 is a factor of 24"). At one point, when she was doing a level where one of the targets was something like 5/4, I said something to the effect of "OK, so you just need a full power and a quarter power" and she said "I never would have thought of it that way!", but she absolutely understood it in the context of the game. Other quick teachable moments like this arose from time to time. I surreptitiously watched her while I gave the impression that I was watching the Phillies game. I was mostly quiet, enjoying watching her mind work, but occasionally I'd say something. For example, after a couple of rounds where she used lots of trial and error to solve the puzzles when there were fractions of different denominators that needed to be added together (though there were no + signs anywhere) I said something like "Oh, you need to get 7/6 and they give you 1/3 which is 2/6 and 1/2 which is 3/6 ." That made perfect sense to her in the context of the game and, after that brief comment at a time when she was primed for it, she began the next level by doing in-her-head conversions to a common denominator of the input fractions so that she could figure out how to combine them into the target fractions.

In addition to bending light beams to power spaceships, you can optionally chose to collect coins (you get to keep them in your trophy room). As I played, I always tried to get the coins, which can make a level quite a bit harder in some cases. My daughter tended to ignore them unless it was really obvious to her how to get them. Occasionally, when she thought she'd missed an easy coin, she'd retry the level immediately upon completion. I expect that after she's made it through all the levels, if she's still interested in playing the game she'll go back and do it again trying to get all the coins. (I discovered after observing this that there's actually a research article on the game's web page at the University of Washington's Center for Game Science about how different players respond to the presence of coins and what educational game designers need to think about when incorporating such "secondary game objectives.")

Equation of a quadratic

This sleek GeoGebra applet by Michael Borcherds provides practice in determining the equations of quadratic functions from their graphs. Some of the generated parabolas are easier to write equations for in factored form while others are easier to do in (h, k) form. All have leading coefficients of 1. After each function you type in, the function you have given is graphed for comparison with the original and you're told how many you've gotten right and the total amount of time it has taken you.

Contour Plots

This is a well-designed tool for creating and exploring contour plots. It has nice customization options and you can save your plots in a wide range of formats. It comes from Bulgaria and Macedonia's Computational Science and Engineering Program.

True/False Quiz on the Probability of Combined Events

This quiz about concepts and notation associated with the probability of combined events (complementary events, mutually exclusive events, and exhaustive events) includes a link to an electronic textbook chapter on the material from CIMT.

The Volume of a Watermelon

To introduce the idea of finding the volume of a solid of revolution, have students think about finding the volume of a watermelon.

Sampling Distributions

My colleague, Julia, who teaches AP Statistics, says this applet from the Simulations and Demonstrations section of the Rice Virtual Lab in Statistics is very effective for helping students to understand the central limit theorem. After they've got the general idea using typical distributions, students enjoy going to the ActivBoard and using the feature that lets you draw your own population distribution.